Branching Brownian Motion 2016
Lecturers: Dr. Anton Klimovsky & Prof. Dr. Anita Winter.
Time & location: Tuesdays, 14:15-16:00 @ WSC-S-U 3.02.
Begin: April 12.
Frequency: 2 hours/week.
Target audience: Master-level Mathematik.
Pre-requisites: Probability Theory II (WT II).
Description
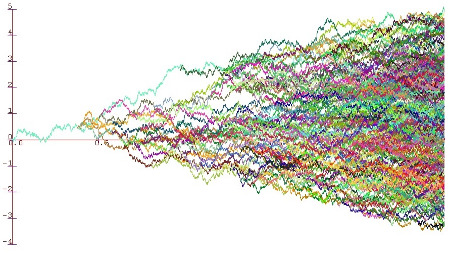
Branching Brownian motion (BBM) is a popular probabilistic model for spatial branching. It combines two classical models: Brownian motion (totally random motion) and the Galton-Watson (GW) process (totally random genealogy).
BBM is a natural spatial embedding of the GW process: Each GW particle gets a position in space and wanders randomly and independently around after its birth. In this seminar, we will especially be interested in the behaviour of the extremal particles (i.e., those having largest/smallest positions) of BBM as time progresses.
Seminar schedule
- 12.04. Themenvergabe und Motivation.
- 26.04. Einführung in Verzweigungsprozesse und Branching Brownian motion (Anita Winter).
- 03.04. F-KPP equation.
- 10.05. Derivative martingale + extreme value theory (Anton Klimovsky).
- 14.06. Feynman-Kac representation + The maximum principle and its applications I. (Tim Kalkmann)
- 21.06. The maximum principle and its applications II.
- 28.06. Estimates on solutions of the linear F-KPP equation + Brownian bridges (Severin Matthes).
- 05.07. Hitting probabilities of curves (Qianli Ma).
- 12.07. Asymptotics of solutions (Geronimo Rojas Barragan).
- Convergence result.
- The extremal process of BBM.